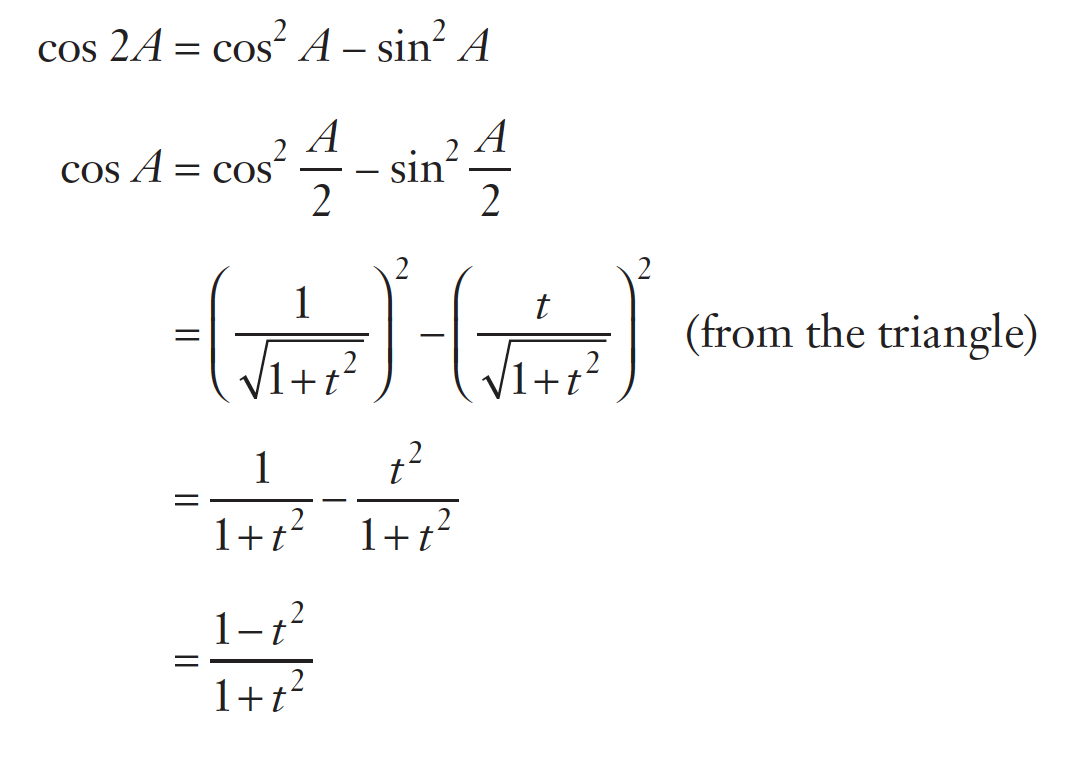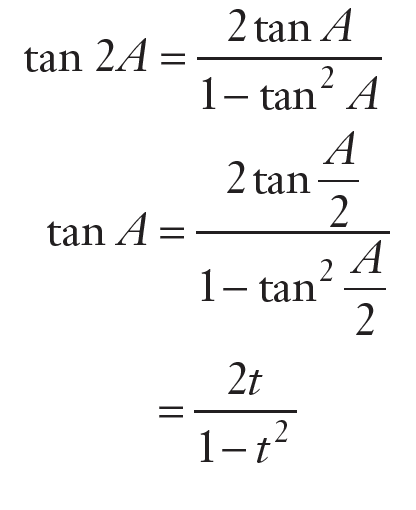Unit Circle

• From advanced mathematics, you may remember the unit circle ¹. This will be important for this module.

  

Sum-Difference Identities

Cosine

$\cos (A\pm B)=\cos (A)\cos (B)\mp \sin (A)\sin (B)$

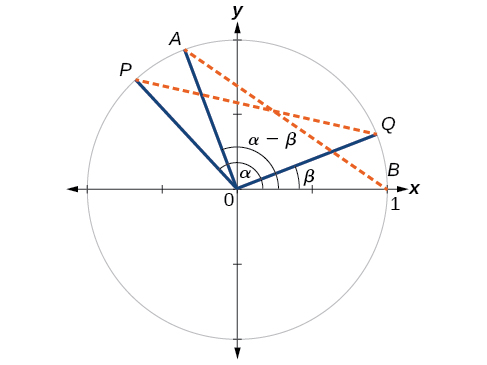
Proof for cosine sum-difference identity

• Consider 2 points ($P$ and $Q$) on the unit circle:
  • Point $P$ is at an angle $\alpha$ from the positive x-axis with coordinates $(\sin (\alpha ),\cos (\alpha ))$
  • Point $Q$ is at an angle of $\beta$ from the positive x-axis, with coordinates $(\sin (\beta ),\cos (\beta ))$
• In this triangle, $\mathrm{\angle }POQ$ will be $\alpha -\beta$

If this hard to visualise, a diagram is coming.

• Let’s make another triangle:
  • Point $A$ is located at an angle of $(\alpha -\beta )$ from the positive x-axis, and has coordinates $(\cos (\alpha -\beta ),\sin (\alpha -\beta ))$
  • Point $B$ is located at $(1,0)$
• If you’ve drawn out the 2 triangles, you will have noticed that $\mathrm{△}AOB$ and $\mathrm{△}POQ$ are rotations of each other, and are therefore congruent, so we can say that $PQ$ and $AB$ are equal

$$\text{Mathematically: }\mathrm{△}AOB\cong \mathrm{△}POQ,\therefore PQ=AB$$

• Now, we can find the distance from $P$ to $Q$ using the Cartesian distance formula: $$d_{PQ}=\sqrt{{(cos\alpha -cos\beta )}^2+{(sin\alpha -sin\beta )}^2}$$ $$=\sqrt{{\cos }^2\alpha -2\cos \alpha \cos \beta +{\cos }^2\beta +{\sin }^2\alpha -2\sin \alpha \sin \beta +{\sin }^2\beta }$$ $$\text{Apply Pythagorean identity and simplify.}$$ $$=\sqrt{({\cos }^2\alpha +{\sin }^2\alpha )+({\cos }^2\beta +{\sin }^2\beta )-2\cos \alpha \cos \beta -2\sin \alpha \sin \beta }$$ $$=\sqrt{1+1-2\cos \alpha \cos \beta -2\sin \alpha \sin \beta }$$ $$=\sqrt{2-2\cos \alpha \cos \beta -2\sin \alpha \sin \beta }$$ $$\text{Similarly, using the distance formula we can find the distance from A to B.}$$ $$d_{AB}=\sqrt{{(\cos (\alpha -\beta )-1)}^2+{(\sin (\alpha -\beta )-0)}^2}$$ $$=\sqrt{{\cos }^2(\alpha -\beta )-2\cos (\alpha -\beta )+1+{\sin }^2(\alpha -\beta )}$$ $$\text{Apply Pythagorean identity and simplify}$$ $$=\sqrt{({\cos }^2(\alpha -\beta )+{\sin }^2(\alpha -\beta ))-2\cos (\alpha -\beta )+1}$$ $$=\sqrt{1-2\cos (\alpha -\beta )+1}$$ $$=\sqrt{2-2\cos (\alpha -\beta )}$$ $$\text{Subtract 2 from both sides and divide both sides by −2.}$$ $$\cos \alpha \cos \beta +\sin \alpha \sin \beta =\cos (\alpha -\beta )$$

Sine

$sin(A\pm B)=\sin A\cos B\pm \cos A\sin B$

Proof for sine sum-difference identity

• Substitute $90°-A$ for $A$ in the cosine sum-difference formula:
  • $\cos ((90°-A)\pm B)=\cos (90°-A)\cos (B)\mp \sin (90°-A)\sin (B)$
  • $\text{Using }\sin (\theta )=\cos (90°-\theta ),\cos (90°-(A\pm B))=\sin (A\pm B)$
  • $=\sin (A)\cos (B)\pm \cos (A)\sin (B)$
  • $\therefore \sin (A\pm B)=\sin (A)\cos (B)\pm \cos (A)\sin (B)$

Tangent

$\tan (A\pm B)=\frac{\sin (A\pm B)}{\cos (A\pm B)}=\frac{\tan (A)\pm \tan (B)}{1\mp \tan (A)tan(B)}$

Proof for tangent sum-difference identity

Honestly I’m not bothered to do it. I’ll probably get carpal tunnel syndrome from all these escape characters.

To prove it yourself, just use $tan\theta =\frac{sin\theta }{cos\theta },$ sub in the $\sin$ and $\cos$ identities, and simplify. It’s not that hard, its just tedious 😕.

- Jackson Taylor

Double Angle Identities

Sine

$\sin (2A)=2\sin (A)\cos (A)$

Proof

$sin(2A)=sin(A+A)$

$=sinAcosA+cosAsinA$

$=2sinAcosA$

Cosine

$cos2A=co{s}^{2}A-si{n}^{2}A$

Proof

$cos(2A)=cos(A+A)$

$=cosAcosA-sinAsinA$

$=co{s}^{2}A-si{n}^{2}A$

Tangent

$tan(2A)=\frac{2\tan (A)}{1-{\tan }^2(A)}$

Proof

$\tan (2A)=\tan (A+A)$

$=\frac{\tan (A)+\tan (A)}{1-\tan (A)\tan (A)}$

$=\frac{2\tan (A)}{1-{\tan }^2(A)}$

Product Identities

• These are just the sum-difference identities, rearranged to find the products of the ratios.

  This means I don’t have to do proofs! Yay!

  - Jackson Taylor

Cosine-Cosine

$cos(A)cos(B)=\frac{1}{2}[cos(A+B)+cos(A-B)]$

Sine-Sine

$sin(A)sin(B)=\frac{1}{2}[cos(A-B)+cos(A+B)]$

Sine-Cosine

$sin(A)cos(B)=\frac{1}{2}[sin(A+B)+sin(A-B)]$

Cosine-Sine

$cos(A)sin(B)=\frac{1}{2}[sin(A+B)-sin(A-B)]$

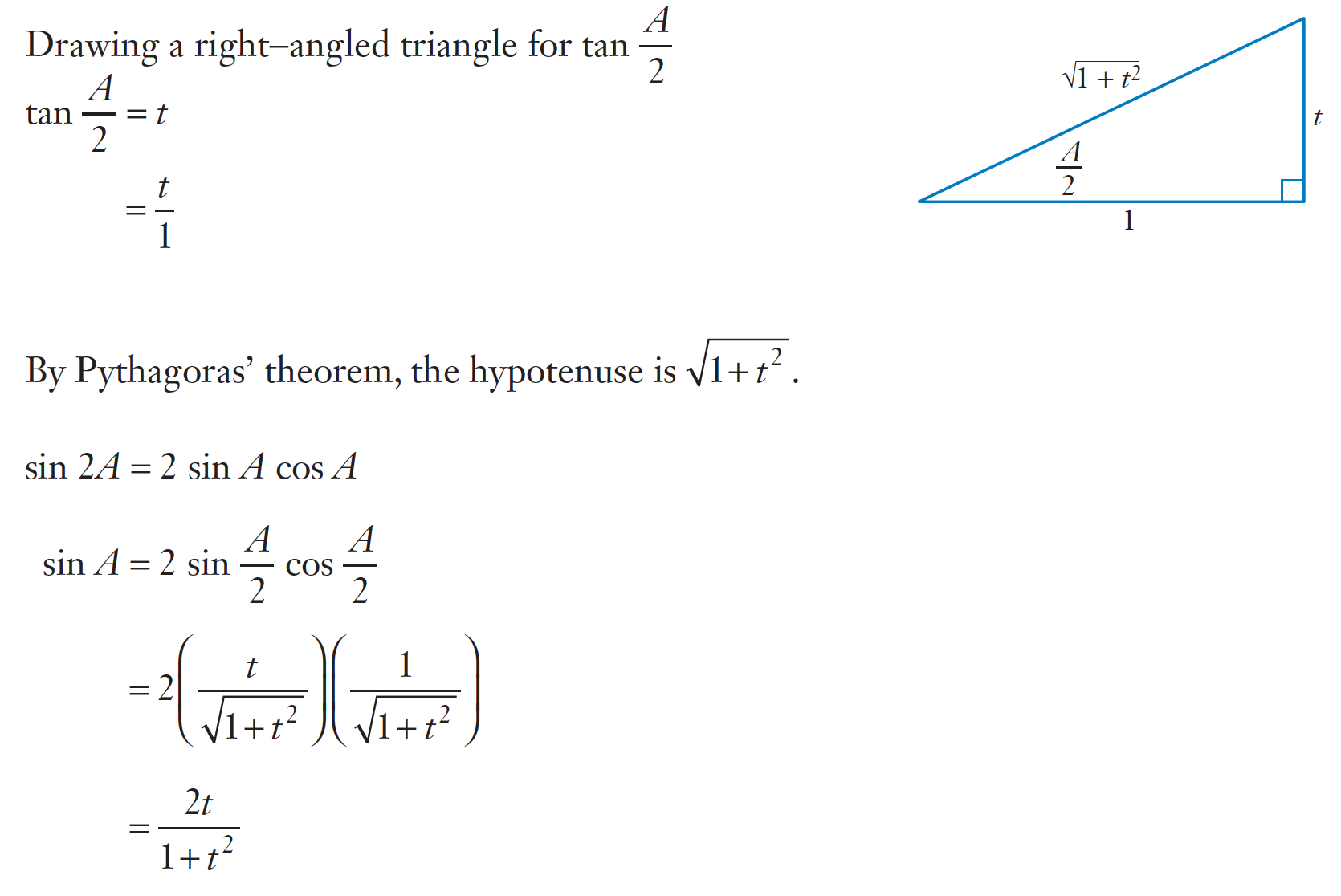
T-Formulae

$t=tan(\frac{A}{2})$

I’m not even bothered to type proofs anymore. Y’all can deal with screenshots.

- Jackson Taylor

• $sinA=\frac{2t}{1+t^2}$

  

• $cosA=\frac{1-t^2}{1+t^2}$

  

• $tanA=\frac{2t}{1-t^2}$

  

Inverse Trigonometric Functions

Notation

Inverse trigonometric functions are notated in one of 2 ways:

• The “arc” prefix:
  • $arcsin(x)$
  • $arccos(x)$
  • $arctan(x)$
  • $arcsec(x)$
  • $arccosec(x)/arccsc(x)$
  • $arccot(x)$
• The inverse ${(}^{-1})$ notation:
  • $si{n}^{-1}(x)$
  • $co{s}^{-1}(x)$
  • $ta{n}^{-1}(x)$
  • $se{c}^{-1}(x)$
  • $cose{c}^{-1}(x)/cs{c}^{-1}(x)$
  • $co{t}^{-1}(x)$

$y={\sin }^{-1}(x)$

$y={\cos }^{-1}(x)$

$y={\tan }^{-1}(x)$

Properties

• ${\sin }^{-1}(-x)=-{\sin }^{-1}(x)$
• $co{s}^{-1}(-x)=\pi -co{s}^{-1}(x)$
• $ta{n}^{-1}(-x)=-ta{n}^{-1}(x)$
• $si{n}^{-1}(x)+co{s}^{-1}(x)=\frac{\pi }{2}$

And we’re done!

That’s all of Extension 1 Trigonometry (for year 11, anyway).

References